About
Articles Related
Minimum Spanning Forest
Formulation
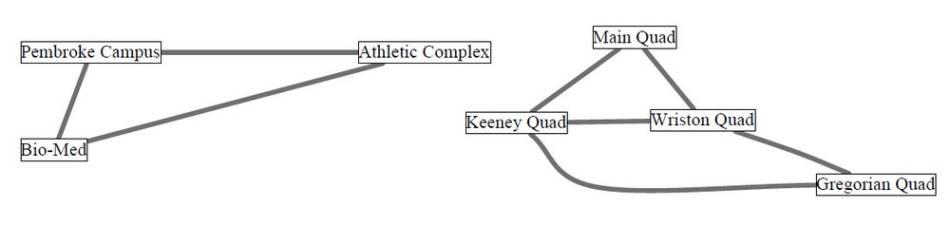
Formulating GF(2) vector: subset {Pembroke, Main, Gregorian} is represented by:
| Pembroke | Athletic | Bio-Med | Main | Keeney | Wriston | Gregorian |
|---|---|---|---|---|---|---|
| 1 | 1 | 1 |
- Edge Representation in term of vectors:
| Edge | Vector | ||||||
|---|---|---|---|---|---|---|---|
| Pembroke | Athletic | Bio-Med | Main | Keeney | Wriston | Gregorian | |
| {Pembroke, Athletic} | 1 | 1 | |||||
| {Pembroke, Bio-Med} | 1 | 1 | |||||
| {Athletic, Bio-Med} | 1 | 1 | |||||
| {Main, Keeney} | 1 | 1 | |||||
| {Main, Wriston} | 1 | 1 | |||||
| {Keeney, Wriston} | 1 | 1 | |||||
| {Keeney, Gregorian} | 1 | 1 | |||||
| {Wriston, Gregorian} | 1 | 1 | |||||
- The vector representing {Keeney, Gregorian} is the sum, for example, of the vectors representing {Keeney, Main }, {Main, Wriston}, and {Wriston, Gregorian}.
- A vector with 1’s in entries x and y is the sum of vectors corresponding to edges that form an x-to-y path in the graph. For instance, the span of the vectors representing {Pembroke, Bio-Med}, {Main, Wriston}, {Keeney, Wriston}, {Wriston, Gregorian }
- contains the vectors corresponding to
{Main, Keeney}, {Keeney, Gregorian}, and {Main, Gregorian}
- but not the vectors corresponding to
{Athletic, Bio-Med } or {Bio-Med, Main}
Algorithm
def Grow(G)
S := 0;
consider the edges in increasing order
for each edge e:
if e’s endpoints are not yet connected
add e to S.
def Grow(V)
S = 0;
repeat while possible:
find a vector v in V not in Span S,
and put it in S.
- Considering edges e of G corresponds to considering vectors v in V
- Testing if e’s endpoints are not connected corresponds to testing if v is not in Span S.
The Grow algorithm for MSF is a specialization of the Grow algorithm for vectors. Same for the Shrink algorithms.
Basis
One kind of basis in a graph G: a set S of edges forming a spanning forest.
- Spanning: for each edge xy in G, there is an x-to-y path consisting of edges of S.
- Independent: no cycle consisting of edges of S
A basis for a graph is a spanning forest. Unique Representation shows that, for each edge xy in the graph,
- there is an x-to-y path in the spanning forest, and
- there is only one such path.